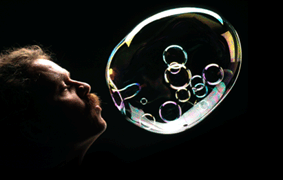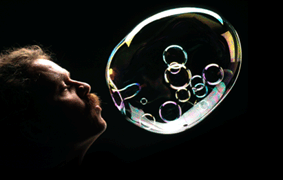SCIENCE
Soap Bubbles are so pure and simple, it's only natural
that kids, physisists and mathematicians are their biggest fans.
I understand a bit about the "Plateau Problems" and
other reasons why bubbles have been of interest to mathematicians
from Isaac Newton to present day scientists working with super computers
but the truth is that I know more about the physics of bubbles than
about the mathematics.
In fact, mathematicians fascinate me partly because I do not understand
them. The language of math is foreign to me; the written form of
the language, equations, is a language written in an unfamiliar alphabet.
But hearing mathematicians speak about bubbles has led me to some
wonderfully abstract thinking. As Alice said in Through the Looking
Glass when she read the nonsense poem Jabberwocky "It seems
very pretty but it is rather hard to understand! Somehow it seems
to fill my head with ideas--only I don't know what they are!"
"My grandfather talked continuously about soap bubbles,
and of course in mathematical terms. I did not understand a
word of what he said."
—Bernhard Caesar Einstein, the grandson
of Albert Einstein |
If you look closely at the suds in the sink the next
time you are doing the dishes you'll be tempted, at first, to agree
that this is a good example of the chaos of nature.
There are so many different shapes to the cells...pyramids, boxes,
mulitsided irregular shapes all stuck together. Look closer...
Bubble walls always join three walls along an edge at three equal
120° angles. The edges always join four edges at a point, the
angle there is always 109° 28' 14".
That apparent chaos is, in fact, a network that is demonstrating
nature's insistence on minimal forms. Nature is always minimizing
but rarely do we see so perfect an example of that tendency as we
do when we look at a soap bubble ... or a cluster of soap bubbles.
Trees branch following these same minimizing principles but trees
are complex living structures that are working to balance several
conflicting needs: spreading out a leaf to gather sunlight, sending
down roots toward moisture, carrying nutrient throughout the large
system ... Look closely and you will notice that the tree branches
always join in three way junctions, so do the roots, so do the veins
within the leaves ... this is a minimal way of networking and nature
always minimizes.
The same is true when you look at star clusters or the patterns made by sections
of a tortoise shell or the scales on a fish or the packing of grains of sand
on a beach ... the principles are those of minimal energy ... but they are
more difficult to discern until you know the many forces at work.
In the case of a soap bubble there is really only one force at work
... the minimizing effects of surface tension on these nearly weightless
fluid forms. The films are, for the most part, thinner than wavelengths
of light (!). There is hardly any mass, therefore, hardly any distorting
effects of gravity in choosing what shapes are assumed. There is
no life force acting to gather nutrition or to spread out in the
sun. They are only trying to minimize and they are fluid so they
can keep moving until they find THE minimal shapes.
 A
single soap bubble in the air is a nearly perfect sphere. The reason
for this is the same reason that planets are spheres, or stars ...
A single force (gravity for the planet and star, the electrical attractions
that appear as surface tension for the bubble) is acting to minimize
the form of the object. A sphere is the most economical shape in
nature, it uses the least amount of surface area to contain a given
volume. A
single soap bubble in the air is a nearly perfect sphere. The reason
for this is the same reason that planets are spheres, or stars ...
A single force (gravity for the planet and star, the electrical attractions
that appear as surface tension for the bubble) is acting to minimize
the form of the object. A sphere is the most economical shape in
nature, it uses the least amount of surface area to contain a given
volume.
If a bubble were any other shape (it is often oval while being blown)
it will keep moving until it finds a spherical shape ... and once
it does, it stops adjusting its shape ... it has arrived at the minimal
and only there will it stabilize.
When two or more bubbles touch they act to share a common wall,
thereby saving material for both. But they don't just settle for
any arrangement where their edges join. They are fluid, they keep
moving until they find the minimal arrangement and that is ... three
walls along an edge joined at 120°, four edges at a point at
109° 28' 14" ... if there were a more economical way to
join they would join in that way ... there isn't.
Bubbles have fascinated physicists and mathematicians for centuries.
Sir Isaac Newton made his own bubble formulas, others preceded and
followed him in efforts to understand their nature. One of the most
useful ways that they have served science is in showing minimal areas
when applied tovarious geometric frames. Oddly, there is no good
mathematics for solving these very basic questions.
If you were to take a ring or hoop and ask what shape would be necessary
to fill the space within the round ring ... you may intuitively guess
at a flat round disc. Any hills or valleys within that disc would
cause the form to have extra surface not needed to fill the ring.
If you were to dip that ring or hoop into soapy water you would get
a film that is a flat round disc.
Now, suppose that you bent the ring here and there into some oddly
curvy shape (but still closed like a ring). Now what is the minimal
shape needed to fill that new wavy ring? We don't know ... and there
is no mathematics to help you settle the question.
 This
is part of an old mathematics puzzle called the Plateau Problems.
Plateau, a Belgian physicists asked questions like these in the 1880s
and many of his questions are still unanswered. It is known that
if you dip that wavy ring into soapy water you would pick up a film
that is the most minimal shape possible ... soap films must minimize,
they have no choice. This
is part of an old mathematics puzzle called the Plateau Problems.
Plateau, a Belgian physicists asked questions like these in the 1880s
and many of his questions are still unanswered. It is known that
if you dip that wavy ring into soapy water you would pick up a film
that is the most minimal shape possible ... soap films must minimize,
they have no choice.
An architect named Otto Frei used this principle to design some
beautiful buildings using the soap films ability to show him minima.
He was therefore able to construct buildings that did not need extra
pillars or other help to hold up the walls or ceiling because he
knew that he was using the most minimal shapes for the construction
of his light weight materials. He knew it was the most minimal because
he tested it with soap films ... mathematics alone was not enough,
he needed the soap bubbles.
School Science Project
Through my website I often receive letters from students who
are working on school projects. Some are very polite and some less
so. Often the students are asking that I simply hand over the information
that they’ve been assigned to discover. I do my best with the
email that I receive but, of course, with so many science projects
going on out there it would be impossible to engage in extensive
dialogue with everyone who writes. I often simply refer them to the
information that others and I have already written and put on their
websites.
But I had a very good exchange with a high school freshman who
used her own initiative as well as my contributions to put together
a nice presentation. I appreciated they way that she approached the
project and the way that she approached me and so I thought that
I would share that exchange with those interested. CLICK
HERE to read the exchange.
Expedition Six
Space Chronicles #8
NASA website article by: ISS Science Officer Don Pettit
http://spaceflight.nasa.gov/station/crew/exp6/spacechronicles8.html
 In
early 2003 Science Officer Pettit, an astronaut aboard the International
Space Station, prepared to experiment with soap bubbles in space.
As you will read in the text, he was distracted from that experiment
when he was surprised to find that he could produce a durable film
with pure water. This was an unanticipated new bit of science. Water
films on the Earth's surface have difficulty forming and are not
at all durable. Many (including me) have assumed that this was the
result of the pull of the surface tension combined with the ready
evaporation of the water when it is unprotected by the layer of soap
at the surface. Now we can see that the pull of gravity played an
even bigger role in destroying the pure water film whenever it did
form (think of the temporary water bubbles that form and collapse
when you run water into your sink or bathtub). In zero gravity (0g)
that force plays no part and the surprisingly thick water films can
deal with the effects of evaporation for a long time (though it does
not appear that film formed into pure water bubbles were nearly as
long-lasting). In
early 2003 Science Officer Pettit, an astronaut aboard the International
Space Station, prepared to experiment with soap bubbles in space.
As you will read in the text, he was distracted from that experiment
when he was surprised to find that he could produce a durable film
with pure water. This was an unanticipated new bit of science. Water
films on the Earth's surface have difficulty forming and are not
at all durable. Many (including me) have assumed that this was the
result of the pull of the surface tension combined with the ready
evaporation of the water when it is unprotected by the layer of soap
at the surface. Now we can see that the pull of gravity played an
even bigger role in destroying the pure water film whenever it did
form (think of the temporary water bubbles that form and collapse
when you run water into your sink or bathtub). In zero gravity (0g)
that force plays no part and the surprisingly thick water films can
deal with the effects of evaporation for a long time (though it does
not appear that film formed into pure water bubbles were nearly as
long-lasting).
It seems as though that nature has a few more surprises up her sleeve
and it's a good idea to keep our eyes open even (or especially) when
looking at phenomena that we believe we already understand.
To see some more of Astronaut Pettit's experiments in space with
water and air bubbles click on this link to Google's video page:
http://video.google.com/videoplay?docid=-7696080327508939266
(if the clip plays and stops, put it on pause until a good bit has
loaded in and then play)
©Tom Noddy and Bubble Magic
P.O. Box 1576 • Santa Cruz, California • 95061 • USA • (831) 426-2230
Site design by
Luckydog Arts
and Design©2006
bubble, bubbles, entertainment, performance, performer,
entertainer ,show, act, magic, soap, blowing, mr. bubbles, bubblemania,
bubbleology, bubblology, science, night club, circus, variete, vaudeville,
vaude, music hall, original, best, first, beautiful, gorgeous, pictures,
photos, tonight, that's incredible museum center, mathematics, physics,
ligh,t reflection, wave, interference, rudi, carrell, sebastian,
paul daniels television, space, unique, detergent, glycerin,
|